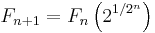Tower of fields

In mathematics, a tower of fields is a sequence of field extensions

F₀ ⊆ F₁ ⊆ ... ⊆ F_n ⊆ ...

The name refers to the fact that such sequences are often written as

$\begin{array}{c}\vdots \\ | \\ F_2 \\ | \\ F_1 \\ | \\F_0. \end{array}$

A tower of fields is called infinite if it is an infinite sequence, otherwise it is called finite.

Examples

$F_{n%2B1}=F_n\left(2^{1/2^n}\right)$

(i.e. F_n+1 is obtained from F_n by adjoining a 2ⁿth root of 2) is an infinite tower.

If p is a prime number the pth cyclotomic tower of Q is obtained by letting F₀ = Q and F_n be the field obtained by adjoining to Q the pⁿth roots of unity. This tower is of fundamental importance in Iwasawa theory.
The Golod–Shafarevich theorem shows that there are infinite towers obtained by iterating the Hilbert class field construction to a number field.

Section 4.1.4 of Escofier, Jean-Pierre (2001), Galois theory, Graduate Texts in Mathematics, 204, Springer-Verlag, ISBN 978-0-387-98765-1